The Black Scholes Merton Model

[responsivevoice_button]

Click here to download the report

The Black Scholes Merton Model, one of the most important concepts in modern financial theory, was developed by Fischer Black, Robert Merton, and Myron Scholes in 1973 to calculate the value of an option contract. The model was based on certain assumptions that assisted in the derivation of the Black-Scholes formula used to calculate the premium paid for an option.

Assumptions

1. The underlying returns are normally distributed
2. The options are European and can only be exercised at the time of maturity
3. The risk-free rate and volatility of the underlying are known and constant
4. Markets are stochastic, and the movements cannot be predicted
5. The underlying does not pay a dividend

What are Option Contracts?

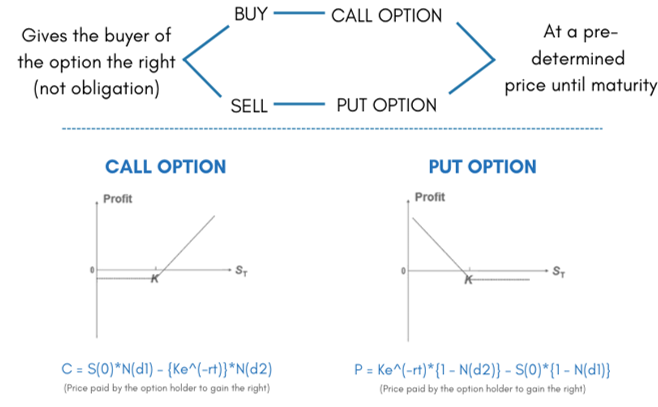
A type of derivative contract which gives the buyer of the option the right but not the obligation to buy or sell an underlying security at a pre-determined price.
The option which gives an individual the right but not the obligation to buy is known as the call option, whereas the one that gives the right but not the obligation to sell is referred to as the put option.
Let us understand in detail what the call and put options are with the help of an example.

Call Option

1. An investor finds a good investment opportunity in stock(X) but is confused and wants to research a bit.
2. Current scenario: The stock price of X is ₹100, and the investor anticipates it to reach ₹120 in 3 months. To profit from the anticipated scenario, the investor uses a call option.
3. The investor then pays a premium of ₹5 and enters into a contract that gives him/her the right but does not oblige him/her to buy the stock X for ₹100 regardless of the prevailing market price.
4. At the end of 3 months, there are two possible scenarios:
   • The price of stock X will be ₹120 or above
   • The price of stock X will be ₹100 or lower
5. If the first scenario persists, the investor will exercise his right and buy the stock X at ₹100, thereby making a minimum profit of ₹15 by selling the stock in the spot market.
6. If the second scenario is true, the investor will not exercise his/her right to buy the stock and would rather bear a loss of the ₹5 premium paid three months ago.
7. Hence, a call option saves the investor from blocking an amount of ₹100 on stocks he/she is not sure about. It serves as a tool that helps in pre-deciding the minimum loss amount or opens up an opportunity to realize unlimited profits.

Put Options

1. An investor completes his research and anticipates that the stock price (X) will fall from the current level. However, he/she is a bit ambiguous about the same.
2. Current scenario: The stock price of X is ₹100, and the investor anticipates it to reach ₹80 in 3 months. To profit from the anticipated scenario, the investor uses a put option.
3. The investor pays a premium of ₹5 and enters into a contract that gives him/her the right but does not oblige him/her to sell the stock X for ₹100 regardless of the prevailing market price.
4. At the end of 3 months, there are two possible scenarios:
   • The price of the stock X will be ₹80 or lower
   • The price of the stock X will be ₹100 or above
5. If the first scenario persists, the investor will exercise his/her right and buy the stock (X) from the market at a lower price and sell the same at the pre-decided price of ₹100 in the spot market, thereby making a minimum profit of ₹15.
6. If the second scenario persists, the investor will not exercise his/her right to buy the stock and would prefer to bear a loss of ₹5 paid as a premium.

Hence, a put option saves the investor from the process of short-selling, wherein he/she will have to pay interest on the proceeds of short-selling while waiting for the stock price to fall and make profits. The put option also serves as a tool to set the maximum amount of loss he/she will have to bear, providing a profit till the stock price reaches zero.

How Does the Black Scholes Merton Model work?

The model hypothesizes that prices of the underlying follow a lognormal distribution with a random walk with constant drift and volatility. Using the assumptions and factoring of a few other variables as inputs, the model derives the formula for calculating the price of a European call and put option.

Inputs required for the model?

The following inputs combined with the assumptions are used to derive an equation that can be used to price a European call and put option:
S = Underlying spot price
K = Exercise price of the option
r = Risk-free rate
t = Time to Maturity
D = Dividend
σ = Volatility
The price of a call option is calculated by subtracting the product of the Net Present Value (NPV) of the strike price and the standard normal probability density function from the product of the stock price and the cumulative standard normal probability density function.

C = S(0)N(d1) – Ke^(-rt)N(d2)

The price of a put option is calculated by subtracting the product of the spot price and standard normal probability density function from the product of the present value of the exercise price and standard normal probability density function.

P = Ke^(-rt){1-N(d2)} – S(0){1-N(d1)}

Where,
d1 = [ln (S/K) + {r + (σ^2/2) *t}] / σ√t =
d2 = d1 – σ√t =
C = Call Option Price
S = Current stock or the underlying price
K = Exercise price of the option
r = Risk-free rate
t = Time to maturity of the option
N(d1) = Call delta
N(d2) = Probability that the call will be in the money
N(-d2) = Probability that the put will be in the money
Besides, the BSM model indicates predictability that the price movements of heavily-traded assets follow a geometric Brownian motion with constant drift and volatility.

Drawbacks of the Black Scholes Merton Model

1. The model assumes risk-free rate and dividends to be constant over the life of the option, which does not happen in reality.
2. The model is only used to value European options; hence, it does not solve the problem of calculating the price to be paid for American options.
3. Volatility is assumed to be constant throughout the option’s life, which is contradictory to the real-life scenario as it fluctuates based on the level of demand and supply.
4. Other assumptions of the model, like no taxes, commission, and transaction costs, lead to results that are deviated from the real world.

Contributor: Team Leveraged Growth